

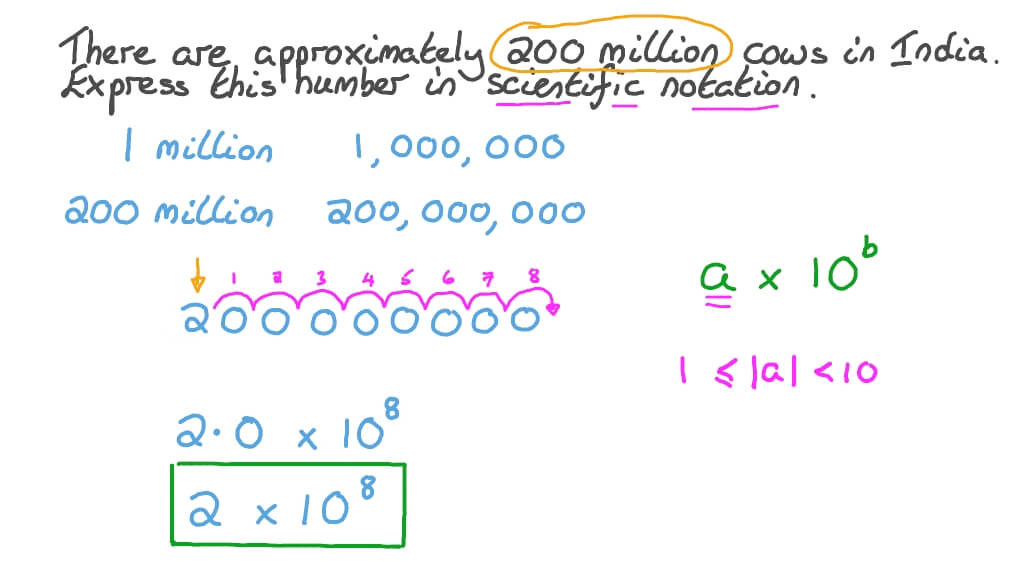
Now, instead of looking at a bunch of zeroes, we can make quantitative comparisons more readily. The examples of large numbers above convert to Scientific Notation as follows (all distances in km):Ħ0 = 6 x 10 1: Washington, D.C to Baltimoreģ700 = 3.7 x 10 3: Washington, D.C. In Scientific Notation, the exponent of 10 defines the direction and the number of places to move the decimal point (to the right for a positive exponent, to the left for a negative exponent). When the exponent of 10 is a negative integer, the exponent defines the number of places to move the decimal point to the left of the 1: When the exponent of 10 is a positive integer, the exponent defines the number of zeroes that come after the 1: (If you have not read the Exponents page, this would be a good time to do so. a number between 1 and darned close to 10) multiplied by a power of 10. In Scientific Notation, any number, no matter how large or small, can be expressed as a number with one digit to the left of the decimal point (i.e. However, beyond that, the numbers all just look “really small” and it gets difficult to tell how much smaller one “really small” number is than another.Įnter Scientific Notation and Powers of Ten. One can maintain a sense of scale through perhaps 3 or 4 places to the right of the decimal point. Here are some approximate dimensions in meters (m):Ġ.0005: Typical diameter of a human egg cellĠ.00001: Typical diameter of a human red blood cellĠ.000000000000001: Diameter of proton or neutron Now let’s take some examples of small numbers. However, beyond a certain number of zeroes, it just looks like a lot of zeroes and all the numbers just look “really big” and it gets difficult to tell how much bigger one “really big” number is than another. One can maintain a sense of scale through a few hundred or perhaps a few thousand kilometers. Let’s look at a few examples, shall we? Here are some approximate distances in kilometers (km):ģ700: Washington, D.C. Numbers larger than 10 tend to get lumped together as “really big numbers.” Numbers less than 1 tend to get lumped together as “really small numbers.” This is surely not a coincidence, given that most of us learn to count on our fingers.
Humans have a predisposition for numbers between 1 and 10.


 0 kommentar(er)
0 kommentar(er)
